Heat Air Moisture Modeling

1. Geometry

Here is an example of the geometry for the heat air moisture modeling.

Figure 1. Geometry of Heat Air Moisture Modeling

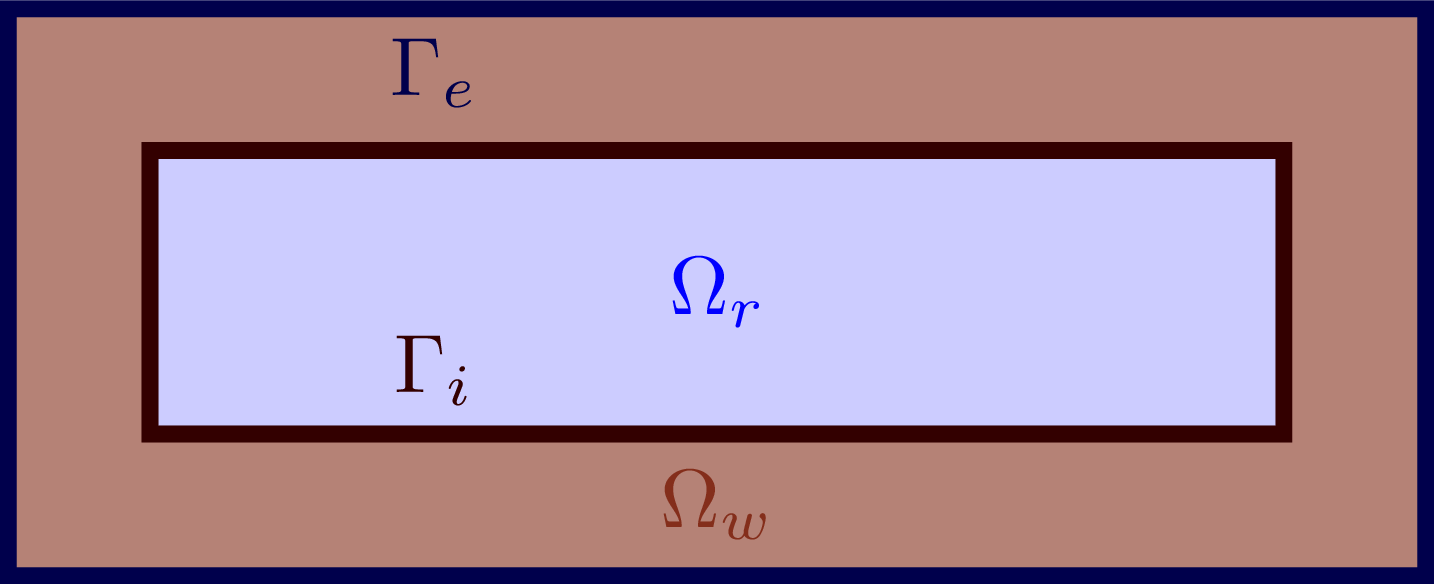
In the following we will use those notations :

$\Omega_r$ is the domain of the room
$\Omega_w$ is the wall domain
$\Gamma_{i}$ is the broder between the room and the wall
$\Gamma_{e}$ is the border between the wall and the exterior

2. Heat transfer modeling

2.1. Equations

Here are the equation of heat transfert in the wall (equation $\ref{hw}$) and in the room (equation $\ref{hr}$), get from the benchmark BESTEST.

\[\begin{align} \left(\rho_m c_m \right)_{\mathrm{eff}} \frac{\partial T_w}{\partial t}+\nabla \cdot\left(-k \nabla T_w - L_{\mathrm{v}} \delta_{\mathrm{p}} \nabla\left(\phi_w p_{\mathrm{sat}}\right)\right) &= 0 \tag{$H_w$}\label{hw}\\ \rho_a c_a \frac{\partial T_r}{\partial t} - h_i \cdot A_w \cdot \left( T_w - T_r \right) - \eta \cdot \rho_a c_a \cdot V_a \cdot \left( T_e - T_r \right) &= H \tag{$H_r$}\label{hr} \end{align}\]

With :

$T_w$ / $T_r$ / $T_e$ [K] is the wall / room / exterior temperature.
$w_w$ / $w_r$ / $w_e$ [K] is the wall / room / exterior absolute humidity.
$\phi_w$ / $\phi_r$ / $\phi_e$ [K] is the wall / room / exterior relative humidity.
$T_e$ [K] is the exterior temperature.
$\phi_w$ [$-$] is the rom relative humidity- $\rho_m$ [$\mathrm{kg} / \mathrm{m}^3$] is the material density.
$c_m$ [$\mathrm{J} / \left(\mathrm{kg} . \mathrm{K} \right)$] is the material thermal mass capacity.
$k$ [$\mathrm{W} \cdot \mathrm{m}^{-1} \cdot \mathrm{K}^{-1}$] is the thermal conductivity.
$\rho_a$ [$\mathrm{kg} / \mathrm{m}^3$] is the air density.
$c_a$ [$\mathrm{J} / \left(\mathrm{kg} \cdot \mathrm{K} \right)$] is the air heat capacity.
$V_a$ [$\mathrm{m}^3$] is the room volume.
$\eta$ [$\mathrm{h}^{-1}$] is the ventilation rate.
$h_i$ (resp. $h_e$) [$\mathrm{W}/ \left(m^2 \cdot K\right)$] is the inside (resp. outside) convective heat transfer coefficient.
$A_w$ [$\mathrm{m}^2$] is the total area of the walls.
$\xi$ [$\mathrm{kg} / \mathrm{m}^{3}$] is the moisture storage capacity.
$L_\mathrm{v}$ [$\text{J}\,\text{kg}^{-1}$] is the latent heat of evaporation.
$\delta_{\mathrm{p}}$ [s] is the vapor permeability.
$p_{\text {sat }}$ [$\mathrm{Pa}$] is the vapor saturation pressure.
$D_{\mathrm{w}}$ [$\mathrm{m}^{2} / \mathrm{s}$] is the moisture diffusivity.
$H$ [$\text{W}$] is the heating power.

The boundary conditions at the border $\Gamma_{i}$ between the wall and the room are :

\[- k\nabla T_w=h_i(T_r-T_{w_{s,i}}) + h_m \cdot L_\mathrm{v} \left( w_r - w_w \right)\]

At the border $\Gamma_{e}$ between the wall and the exterior the conditions are :

\[- k\nabla T_w=h_e(T_e-T_{w_{s,e}}) + h_m \cdot L_\mathrm{v} \left( w_e - w_w \right)\]

2.2. Variational problem

The solve this problem, we use a product of spaces

$X_h$ corresponding to the wall
$W_h$ corresponding to the room : as we consider the temperature as a constant on the room, we take in Feel++ the space Pch<0>. This space corresponds to the piecewise-continous functions which are constant on each element of the mesh : there are therefore constant on all the domain. So, the space Pch<0> is equivalent to $\RR$.

Equation $\ref{hw}$ :

Let $v$ be a test function associated to the wall space. As done before, we linearize the problem with this approxmation :

\[\frac{\partial T_w}{\partial t}\approx\frac{T_w^{n+1}-T_w^n}{\Delta t}\]

The equation $\ref{hw}$ become :

\[\int_{\Omega_w}\left(\rho_m^n c_m^n \right) \frac{T_w^{n+1}}{\Delta t}v + \int_{\Omega_w}\nabla \cdot\left(-k^n \nabla T_w^{n+1} - L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \nabla\left(\phi_w^{n+1} p_{\mathrm{sat}}^n\right)\right)v = \int_{\Omega_w}\left(\rho_m^n c_m^n \right)\frac{T_w^n}{\Delta t} v\]

It leads to, after a partial integration :

\[\begin{equation} \int_{\Omega_w}(\rho_mc_m)^n \frac{T_w^{n+1}}{\Delta t}v + \int_{\Omega_w} \left(k^n \nabla T_w^{n+1} + L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \nabla\left(\phi_w^{n+1} p_{\mathrm{sat}}^n\right)\right) \nabla v - \int_{\partial\Omega_w} \left(\left((-k^n \nabla T_w^{n+1} - L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \nabla\left(\phi_w^{n+1} p_{\mathrm{sat}}^n\right)\right)\cdot n\right)v = \int_{\Omega_w}(\rho_m c_m)^n\frac{T_w^n}{\Delta t}v \end{equation}\]

Which is equivalent, after appying the boundary conditions, as $\partial\Omega_w = \Gamma_{e}\cup \Gamma_{i}$

\[\begin{align} \int_{\Omega_w}(\rho_mc_m)^n \frac{T_w^{n+1}}{\Delta t}v + \int_{\Omega_w} \left(k^n \nabla T_w^{n+1}\right)\nabla v + \\ \int_{\Omega_w} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right) \nabla v + \int_{\Omega_w} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n p_{\mathrm{sat}}^n \nabla\left(\phi_w^{n+1}\right)\right) \nabla v \\ - \int_{\Gamma_{i}} \left((-k^n \nabla T_w^{n+1})\cdot n\right) v - \int_{\Gamma_{e}} \left((-k^n \nabla T_w^{n+1})\cdot n\right) v \\ - \int_{\Gamma_{i}} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)\cdot n\right)v - \int_{\Gamma_{e}} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)\cdot n\right)v \\ - \int_{\Gamma_i} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n p_{\mathrm{sat}}^n \nabla\left(\phi_w^{n+1}\right)\right)\cdot n\right)v - \int_{\Gamma_e} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)\cdot n\right)v \\ = \int_{\Omega_w}(\rho_m c_m)^n\frac{T_w^n}{\Delta t}v \end{align}\]

\[\begin{align} \int_{\Omega_w}(\rho_mc_m)^n \frac{T_w^{n+1}}{\Delta t}v + \int_{\Omega_w} \left(k^n \nabla T_w^{n+1}\right)\nabla v + \\ \int_{\Omega_w} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right) \nabla v + \int_{\Omega_w} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n p_{\mathrm{sat}}^n \nabla\left(\phi_w^{n+1}\right)\right) \nabla v \\ - \int_{\Gamma_{i}} (h_i(T_r^{n+1}-T_w^{n+1}) + h_mL_\mathrm{v}(w_r^{n+1} - w_w^{n+1})) v + \int_{\Gamma_{e}} (h_eT_w^{n+1} + h_m L_\mathrm{v} w_w^{n+1}) v \\ - \int_{\Gamma_{i}} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)v - \int_{\Gamma_{e}} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)v \\ - \int_{\Gamma_i} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n p_{\mathrm{sat}}^n \nabla\left(\phi_w^{n+1}\right)\right)v - \int_{\Gamma_e} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)v \\ = \int_{\Omega_w}(\rho_m c_m)^n\frac{T_w^n}{\Delta t}v + \int_{\Gamma_e} \left(h_eT_e^{n+1} + h_mw_e^{n+1}\right)v \end{align} \tag{$H_{w,\mathrm{disc}}$}\label{hwd}\]

Equation $\ref{hr}$ :

We apply the same Euler scheme on $T_r$, the equation $\ref{hr}$ becomes :

\[\left(\frac{\rho_ac_p}{\Delta t} + h_iA_w + \eta\rho_ac_a V_a\right)T_r^{n+1} - h_iA_wT_w^{n+1} = H^{n+1} + \frac{\rho_ac_p}{\Delta t} T_r^n + \eta\rho_a c_a V_a T_e^{n+1}\]

This equation gives this variational problem :

Find $T_r^{n+1}\in\mathbb{R}$ such as $\forall u\in \RR$ :

\[\int_{\Omega_r}\left(\left(\frac{\rho_ac_a}{\Delta t} + h_iA_w + \eta\rho_ac_a V_a\right)T_r^{n+1}\right)u - \int_{\Omega_r} h_iA_wT_w^{n+1} u = \int_{\Omega_r}\left(H^{n+1} + \frac{\rho_ac_a}{\Delta t} T_r^n + \eta\rho_a c_a V_a T_e^{n+1}\right) u \tag{$H_{r,\mathrm{disc}}$}\label{hrd}\]

The variational problem is : find $T^{n+1}=(T_w^{n+1},T_r^{n+1})\in X_h\times W_h$ such as forall test functions $V=(v,u)$,

\[A(T^{n+1},V) = f(V)\]

with :

\(A(T^{n+1},V) = \begin{bmatrix} \begin{pmatrix} \displaystyle\int_{\Omega_w}(\rho_mc_m)^n \frac{T_w^{n+1}}{\Delta t}v + \int_{\Omega_w} \left(k^n \nabla T_w^{n+1}\right)\nabla v + \int_{\Omega_w} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right) \nabla v + \int_{\Omega_w} \left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n p_{\mathrm{sat}}^n \nabla\left(\phi_w^{n+1}\right)\right) \nabla v \\ - \displaystyle\int_{\Gamma_{i}} \left(-h_i T_w^{n+1} - h_mL_\mathrm{v}(w_w^{n+1})\right) v + \int_{\Gamma_{e}} \left(h_eT_w^{n+1} + h_m L_\mathrm{v} w_w^{n+1}\right) v - \int_{\Gamma_{i}} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)\right)v \\ - \displaystyle\int_{\Gamma_{e}} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)\right)v - \int_{\Gamma_i} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n p_{\mathrm{sat}}^n \nabla\left(\phi_w^{n+1}\right)\right)\right)v - \int_{\Gamma_e} \left(\left(L_{\mathrm{v}}^n \delta_{\mathrm{p}}^n \phi_w^{n+1} \nabla\left(p_{\mathrm{sat}}^n\right)\right)\right)v \end{pmatrix} & - \displaystyle\int_{\Gamma_{i}} \left(h_i T_r^{n+1} + h_mL_\mathrm{v}w_r^{n+1}\right) v \\ - \displaystyle\int_{\Omega_r} h_iA_wT_w^{n+1} u & \displaystyle\int_{\Omega_r}\left(\left(\frac{\rho_ac_a}{\Delta t} + h_iA_w + \eta\rho_ac_a V_a\right)T_r^{n+1}\right)u \end{bmatrix}\)
$f(V) = \begin{bmatrix} \displaystyle\int_{\Omega_w}(\rho_m c_m)^n\frac{T_w^n}{\Delta t}v + \int_{\Gamma_e} \left(h_eT_e^{n+1} + h_mw_e^{n+1}\right)v \\ \displaystyle\int_{\Omega_r}\left(H^{n+1} + \frac{\rho_ac_a}{\Delta t} T_r^n + \eta\rho_a c_a V_a T_e^{n+1}\right) u\end{bmatrix}$

The matrix $A$ represents the form such as the rows correspond to test functions and the columns correspond to the trial function. In our case, this like that :

$\begin{matrix} w \text{ test} \\w \text{ trial}\end{matrix}$

$\begin{matrix} w \text{ test} \\r \text{ trial}\end{matrix}$

$\begin{matrix} r \text{ test} \\w \text{ trial}\end{matrix}$

$\begin{matrix} r \text{ test} \\r \text{ trial}\end{matrix}$

3. Moisture transfer modeling

Here are the equation of heat transfert in the wall (equation $\ref{mw}$) and in the room (equation $\ref{mr}$), get from the benchmark BESTEST.

3.1. Equations

\[\begin{align} \xi\frac{\partial\phi_w}{\partial t} + \nabla\cdot\left(-\xi D_\mathrm{w}\nabla\phi_w-\delta_\mathrm{p}\nabla\left(\phi_wp_\mathrm{sat}\right)\right) = 0 \tag{$M_w$}\label{mw} \\ V_a\frac{\partial w_r}{\partial t} = \eta V_a\left(w_e-w_r\right) + \dot{m}_\mathrm{gen} + h_mA_w\left(w_w-w_r\right) \tag{$M_r$}\label{mr} \end{align}\]

With those boundary conditions :

\[\begin{cases} -\xi D_\mathrm{w}\nabla \phi_w - \delta_\mathrm{p}\nabla(\phi_wp_\mathrm{sat}) = h_{m,i}(w_r-w_w)\qquad \text{on }\Gamma_i\\ -\xi D_\mathrm{w}\nabla \phi_w - \delta_\mathrm{p}\nabla(\phi_wp_\mathrm{sat}) = h_{m,e}(w_e-w_w)\qquad \text{on }\Gamma_i \end{cases}\]

3.2. Variational problem

Wall equation $\ref{mw}$ :

We do the same process as before to get the variational formulation, with a test function $v$ :

\[\int_{\Omega_w}\xi^n\frac{\phi_w^{n+1}}{\Delta t}v + \int_{\Omega_w}\nabla\cdot\left(-\xi^n D_\mathrm{w}^n\nabla\phi_w^{n+1}-\delta_\mathrm{p}^n\nabla\left(\phi_w^{n+1} p_\mathrm{sat}^n\right)\right)v = \int_{\Omega_w}\xi^n\frac{\phi_w^n}{\Delta t}v\]

\[\int_{\Omega_w}\xi^n\frac{\phi_w^{n+1}}{\Delta t}v + \int_{\Omega_w}\left(\xi^n D_\mathrm{w}^n\nabla\phi_w^{n+1}+\delta_\mathrm{p}^n\nabla\left(\phi_w^{n+1} p_\mathrm{sat}^n\right)\right)\cdot\nabla v - \int_{\Gamma_i\cup\Gamma_e}\left(\left(\xi^n D_\mathrm{w}^n\nabla\phi_w^{n+1}+\delta_\mathrm{p}^n\nabla\left(\phi_w^{n+1} p_\mathrm{sat}^n\right)\right)\cdot n\right) v = \int_{\Omega_w}\xi^n\frac{\phi_w^n}{\Delta t}v\]

\[\begin{align} \int_{\Omega_w}\xi^n\frac{\phi_w^{n+1}}{\Delta t}v + \int_{\Omega_w}\left(\xi^n D_\mathrm{w}^n\nabla\phi_w^{n+1}+\delta_\mathrm{p}^n\nabla\left(\phi_w^{n+1} p_\mathrm{sat}^n\right)\right)\cdot\nabla v \\ + \int_{\Gamma_i}(h_{m,i}\left(w_r^{n+1}-w_w^{n+1}\right) v - \int_{\Gamma_e}\left(h_{m,e}w_w\right) v\\ = \int_{\Omega_w}\xi^n\frac{\phi_w^n}{\Delta t}v - \int_{\Gamma_e} (h_{m,e}w_e)v \end{align} \tag{$M_{w,\text{disc}}$}\]

Room equation $\ref{mr}$ :

The equation $\ref{mr}$ become, for a test function $u\in\RR$ :

\[\int_{\Omega_r}\left(\frac{V_a}{\Delta t} + \eta V_a + h_m A_w\right)w_r^{n+1} u - \int_{\Omega_r}h_m A_w w_w^{n+1} u = \int_{\Omega_r}\left(\eta V_a w_e^{n+1} + \dot{m}_\mathrm{gen}\right)u \tag{$M_{r,\text{disc}}$}\label{eq:m_disc}\]

The variational formulation for the moisture problem is :

Find $\phi^{n+1}=(\phi_w^{n+1},\phi_r^{n+1})\in X_h\times W_h$ such as forall test function $V = (v,u)$ :

\[B(\phi^{n+1},V) = g(V)\]

with :

$B(\phi^{n+1},V) = \begin{bmatrix} \begin{pmatrix}\displaystyle\int_{\Omega_w}\xi^n\frac{\phi_w^{n+1}}{\Delta t}v + \int_{\Omega_w}\left(\xi^n D_\mathrm{w}^n\nabla\phi_w^{n+1} + \delta_\mathrm{p}^n\nabla\left(\phi_w^{n+1} p_\mathrm{sat}^n\right)\right)\cdot\nabla v \\ - \displaystyle\int_{\Gamma_i}\left(h_{m,i} w_w^{n+1}\right) v - \int_{\Gamma_e}\left(h_{m,e}w_w\right) v \end{pmatrix} & \displaystyle\int_{\Gamma_i}\left(h_{m,i} w_r^{n+1}\right) v \\ - \displaystyle\int_{\Omega_r}h_m A_w w_w^{n+1} u & \displaystyle\int_{\Omega_r}\left(\frac{V_a}{\Delta t}+\eta V_a + h_m A_w\right)w_r^{n+1} u \end{bmatrix}$
$g(V) = \begin{bmatrix} \displaystyle\int_{\Omega_w}\xi^n\frac{\phi_w^n}{\Delta t}v - \int_{\Gamma_e} (h_{m,e}w_e)v \\ \displaystyle\int_{\Omega_r}\left(\eta V_a w_e^{n+1} + \dot{m}_\mathrm{gen}\right)u \end{bmatrix}$

4. Resolution wih Feel++

To solve such a problem with Feel++, we use product spaces. Mode details can be found in the internship report.